Notebook source code:
examples/kepler-c/kepler.ipynb
Run the notebook yourself on binder

Kepler (with conjugate point test)¶


Minimum time control of the Kepler equation (CNES / TAS / Inria / CNRS collaboration):
\[t_f \to \min,\]
\[\ddot{q} = -\mu\frac{q}{|q|^3}+\frac{u}{m}\,,\quad t \in [0,t_f],\]
\[\dot{m} = -\beta|u|,\quad |u| \leq T_{\mathrm{max}}.\]
Fixed initial and final Keplerian orbits (fixed final longitude for conjugate point test).
Initializations¶
[1]:
import numpy as np
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import Axes3D
import time
from nutopy import nle
from nutopy import tools
from nutopy import ocp
ctmax = (3600**2) / 1e6 # Conversion from Newtons
mass0 = 1500. # Initial mass of the spacecraft
beta = 1.42e-02 # Engine specific impulsion
mu = 5165.8620912 # Earth gravitation constant
t0 = 0. # Initial time (final time is free)
x0 = np.array([ 11.625, 0.75, 0., 6.12e-02, 0., 3.14159265358979 ]) # Initial state (fixed initial longitude)
xf_fixed = np.array([ 42.165, 0., 0., 0., 0., 0. ]) # Final state (fixed final longitude, see below for each tmax)
n = len(x0)
# tmax = 60 Newtons
#tmax = ctmax * 60.; tf = 15.2055; p0 = -np.array([ .361266, 22.2412, 7.87736, 0., 0., -5.90802 ]); xf_fixed[5] = 10.; N = 1000; nc = 5
# tmax = 9 Newtons
#tmax = ctmax * 9; tf = 93.272; p0 = -np.array([ -4.743728521400288e+00, -7.171314803577050e+01, -2.750468259255777e+00, 4.505679920818070e+01, -3.026794469744788e+00, 2.248091027899508e+00 ]); xf_fixed[5] = 36.; N = 1000; nc = 5
# tmax = 6 Newtons
tmax = ctmax * 6.; tf = 1.32e2; p0 = -np.array([ -4.743728539366440e+00, -7.171314869854240e+01, -2.750468309804530e+00, 4.505679923365745e+01, -3.026794475592510e+00, 2.248091067047670e+00 ]); xf_fixed[5] = 51.; N = 1000; nc = 5
p0 = p0 / np.linalg.norm(p0) # Normalization |p0|=1 for free final time
y = np.hstack((p0, tf)) # initial guess, y = (p0, tf)
Hamiltonian (fortran wrapper)¶
The first and second derivatives of the code are generated by Tapenade. Important: arguments dx and dx0 (and dp and dp0) have been exchanged; the Tapenade generated signature was
SUBROUTINE HFUN_D_D(t, x, xd0, xd, p, pd0, pd, tmax, mass0, beta, mu, h, hd, hdd, n)
while the corrected one is
SUBROUTINE HFUN_D_D(t, x, xd, xd0, p, pd, pd0, tmax, mass0, beta, mu, h, hd, hdd, n)
This ensures that the signature matches what is expected by @tensorize: variations, up to any order, come as x, dx, d2x, d3x, ...
[2]:
!python -m numpy.f2py -c hfun.f90 -m hfun > /dev/null 2>&1
!python -m numpy.f2py -c hfun_d.f90 -m hfun_d > /dev/null 2>&1
!python -m numpy.f2py -c hfun_d_d.f90 -m hfun_d_d > /dev/null 2>&1
from hfun import hfun
from hfun_d import hfun_d
from hfun_d_d import hfun_d_d
hfun = tools.tensorize(hfun_d, hfun_d_d, tvars=(2, 3), full=True)(hfun)
h = ocp.Hamiltonian(hfun)
f = ocp.Flow(h)
Shooting function¶
[3]:
def dshoot(t0, dt0, x0, dx0, p0, dp0, tf, dtf, next=False):
(xf, dxf), (pf, dpf) = f((t0, dt0), (x0, dx0), (p0, dp0), (tf, dtf), tmax, mass0, beta, mu)
s = np.zeros(7) # code duplication and full=True
s[0:6] = xf[0:6] - xf_fixed # fixed final longitude
s[6] = p0[0]**2 + p0[1]**2 + p0[2]**2 + p0[3]**2 + p0[4]**2 + p0[5]**2 - 1.
ds = np.zeros(7)
ds[0:6] = dxf[0:6]
ds[6] = 2*p0[0]*dp0[0] + 2*p0[1]*dp0[1] + 2*p0[2]*dp0[2] + 2*p0[3]*dp0[3] + 2*p0[4]*dp0[4] + 2*p0[5]*dp0[5]
if not next: return s, ds
else: return s, ds, ((tf, dtf), (xf, dxf), (pf, dpf), None)
@tools.vectorize(vvars=(1, 2, 3))
@tools.vectorize(vvars=(4,), next=True)
@tools.tensorize(dshoot, full=True)
def shoot(t0, x0, p0, tf, next=False):
"""s = shoot(t0, x0, p0, tf)
Shooting function associated with h
"""
xf, pf = f(t0, x0, p0, tf, tmax, mass0, beta, mu)
s = np.zeros(7)
s[0:6] = xf[0:6] - xf_fixed
s[6] = p0[0]**2 + p0[1]**2 + p0[2]**2 + p0[3]**2 + p0[4]**2 + p0[5]**2 - 1.
if not next: return s
else: return s, (tf, xf, pf, None)
def jshoot(t0, x0, p0, tf, dx0=None, dp0=None):
"""jac = jshoot(t0, x0, p0, tf)
Jacobian of shooting function wrt. p0 and tf
Vectorized in tf"""
n = x0.shape[0]
if dx0 is None: dx0 = np.zeros((n, n))
if dp0 is None: dp0 = np.eye(n)
if isinstance(tf, list): vec = True
else: vec = False; tf = [ tf ]
N = len(tf)
jac = np.zeros((n+1, N, n+1))
_, jac[0:n, :, :] = shoot([ t0 for i in range(0, n) ], [ (x0, dx0[i, :]) for i in range(0, n) ], [ (p0, dp0[i, :]) for i in range(0, n) ], tf)
_, jac[ n, :, :] = shoot(t0, x0, p0, [ (tf[j], 1.) for j in range(0, N) ])
jac = np.transpose(jac, (1, 2, 0)) # axis 0: d/dp0 or dtf (n+1), 1: time vectorization (N), 2: dim of shooting value (n+1)
if vec: return jac
else: return jac[0]
Solve¶
[4]:
dfoo = lambda y, dy: shoot(t0, x0, (y[:-1], dy[:-1]), (y[-1], dy[-1]))
foo = lambda y: shoot(t0, x0, y[:-1], y[-1])
foo = tools.tensorize(dfoo, full=True)(foo)
nleopt = nle.Options(SolverMethod='hybrj', Display='on', TolX=1e-8)
et = time.time(); sol = nle.solve(foo, y, df=foo, options=nleopt); y_sol = sol.x; et = time.time() - et
print('Elapsed time:', et)
print('y_sol =', y_sol)
print('foo =', foo(y_sol))
Calls |f(x)| |x|
1 6.301595264456300e+00 1.320037878244408e+02
2 2.340071357355818e+00 1.400203658343002e+02
3 2.400735354119709e+00 1.424118392572792e+02
4 1.013403473233678e+00 1.421946981533602e+02
5 2.682895030563751e-01 1.417788071311752e+02
6 7.955498964446011e-01 1.414641043538589e+02
7 1.470638840331741e-01 1.418509100088929e+02
8 4.973211617416135e-02 1.416148603181079e+02
9 1.219551393639842e-01 1.416431105728263e+02
10 3.072632648243853e-02 1.416205384607907e+02
11 8.653617036143087e-03 1.416150551345118e+02
12 3.801480838671332e-03 1.416129848677609e+02
13 2.860024581815136e-03 1.416130187627090e+02
14 1.662360458139482e-03 1.416132110526509e+02
15 9.636113150738866e-04 1.416133352433271e+02
16 1.105532175256260e-03 1.416136891521962e+02
17 1.261272792993838e-05 1.416135006400824e+02
18 1.447548770918053e-06 1.416135019292940e+02
19 8.703313705554255e-08 1.416135017425102e+02
20 5.650491653709091e-08 1.416135016952747e+02
21 6.912864801895259e-09 1.416135016683978e+02
Results of the nle solver method:
xsol = [ 7.06313513e-02 5.05725929e-01 2.14393994e-02 -8.58637026e-01
3.87194628e-02 6.00912505e-03 1.41609971e+02]
f(xsol) = [ 3.00909875e-09 3.37502665e-11 2.50889895e-09 -9.43460900e-11
-4.54824498e-10 -5.67042235e-09 2.60490518e-10]
nfev = 21
njev = 1
status = 1
success = True
Successfully completed: relative error between two consecutive iterates is at most TolX.
Elapsed time: 9.6191086769104
y_sol = [ 7.06313513e-02 5.05725929e-01 2.14393994e-02 -8.58637026e-01
3.87194628e-02 6.00912505e-03 1.41609971e+02]
foo = [ 3.00909875e-09 3.37502665e-11 2.50889895e-09 -9.43460900e-11
-4.54824498e-10 -5.67042235e-09 2.60490518e-10]
Plots¶
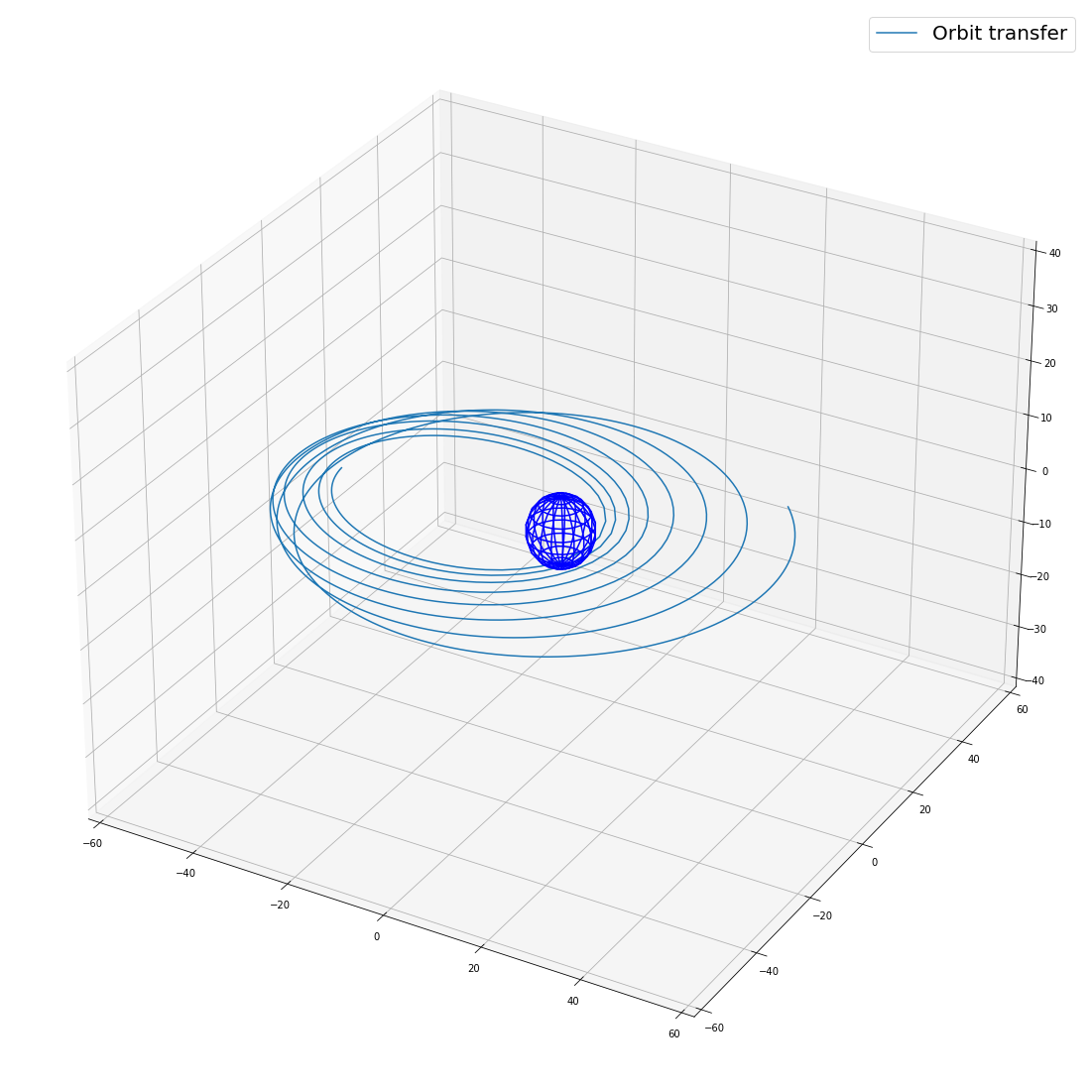
[5]:
p0 = y_sol[:-1]
tf = y_sol[-1]
tspan = list(np.linspace(0, tf, N+1))
xf, pf = np.array( f(t0, x0, p0, tspan, tmax, mass0, beta, mu) )
P = xf[:, 0]
ex = xf[:, 1]
ey = xf[:, 2]
hx = xf[:, 3]
hy = xf[:, 4]
L = xf[:, 5]
cL = np.cos(L)
sL = np.sin(L)
W = 1+ex*cL+ey*sL
Z = hx*sL-hy*cL
C = 1+hx**2+hy**2
q = np.zeros((N+1, 3))
q[:, 0] = P*( (1+hx**2-hy**2)*cL + 2*hx*hy*sL ) / (C*W)
q[:, 1] = P*( (1-hx**2+hy**2)*sL + 2*hx*hy*cL ) / (C*W)
q[:, 2] = 2*P*Z / (C*W)
plt.rcParams['legend.fontsize'] = 20
plt.rcParams['figure.figsize'] = (20, 20)
fig1 = plt.figure()
ax = fig1.gca(projection='3d')
ax.set_xlim3d(-60, 60) # ax.axis('equal') not supported
ax.set_ylim3d(-60, 60)
ax.set_zlim3d(-40, 40)
u, v = np.mgrid[ 0:2*np.pi:20j, 0:np.pi:10j ]
r = 6.378 # Earth radius (in Mm)
x1 = r*np.cos(u)*np.sin(v)
x2 = r*np.sin(u)*np.sin(v)
x3 = r*np.cos(v)
ax.plot_wireframe(x1, x2, x3, color='b')
ax.plot(q[:, 0], q[:, 1], q[:, 2], label='Orbit transfer')
#ax.quiver(q[:, 0], q[:, 1], q[:, 2], u, v, w, length=0.1, normalize=True)
ax.legend()
[5]:
<matplotlib.legend.Legend at 0x7ff343e58d10>
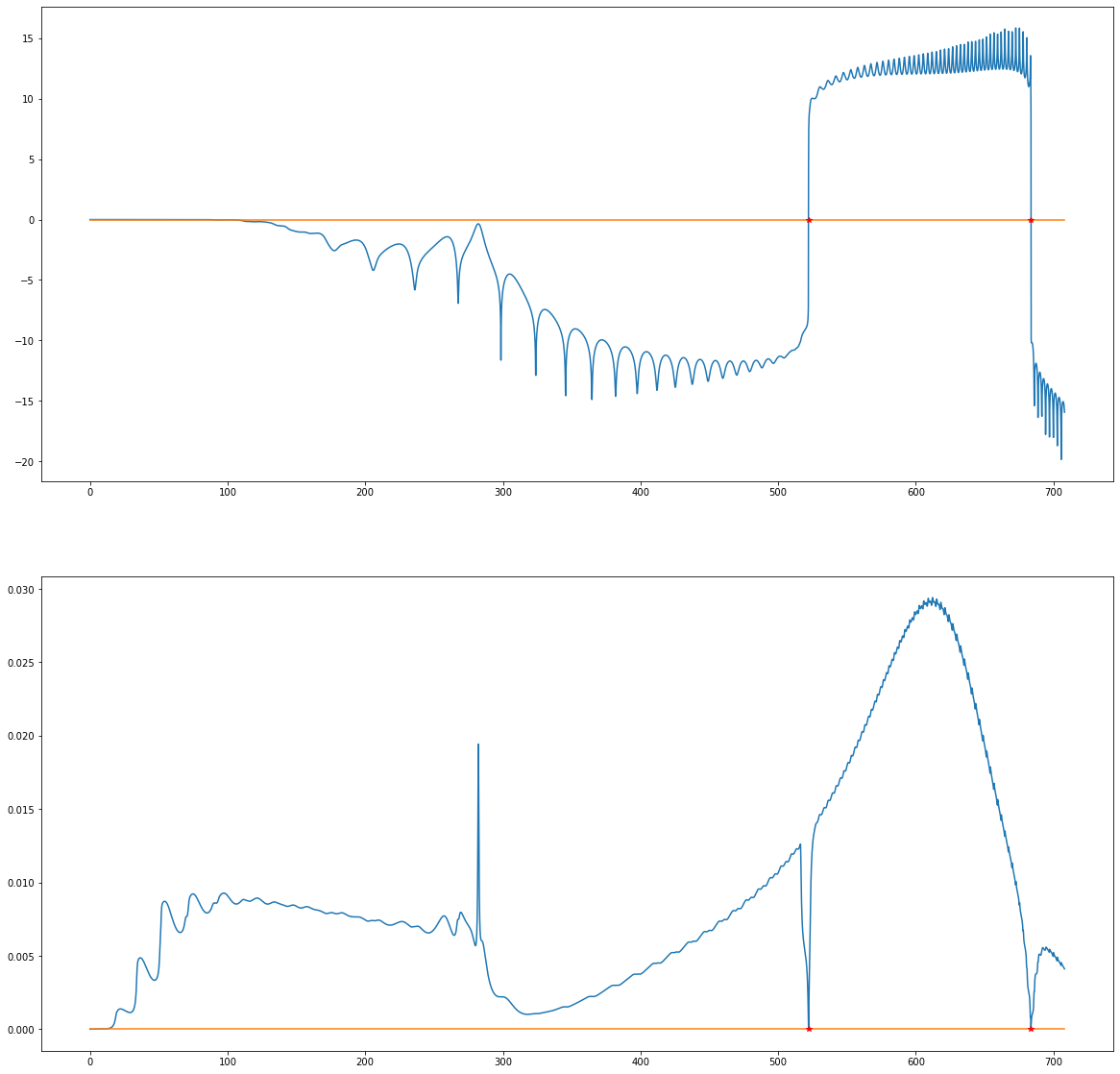
Conjugate point computation¶
[6]:
N = nc*N
tspan = list(np.linspace(0, nc*tf, N))
jac = jshoot(t0, x0, p0, tspan)
det = [ np.linalg.det(jac[i]) for i in range(0, N) ]
sn = [ np.linalg.svd(jac[i])[1][-1] for i in range(0, N) ]
fig2 = plt.figure()
ax21 = plt.subplot(2, 1, 1)
ax21.plot(tspan, np.arcsinh(det))
ax21.plot([ tspan[0], tspan[-1]], [ 0, 0 ])
ax22 = plt.subplot(2, 1, 2)
ax22.plot(tspan, sn)
ax22.plot([ tspan[0], tspan[-1]], [ 0, 0 ])
itc = [ i for i in range(0, N-1) if det[i]*det[i+1] < 0 ]
tspan = np.array(tspan)
tc = np.array(tspan[itc])
dx0 = np.zeros((n, n))
dp0 = np.eye(n)
nleopt = nle.Options(Display='on', TolX=1e-8)
for k in range(0, len(itc)):
dxk, dpk = np.array( f([ t0 for i in range(0, n) ], [ (x0, dx0[i, :]) for i in range(0, n) ], [ (p0, dp0[i, :]) for i in range(0, n) ], tc[k], tmax, mass0, beta, mu) )
xk = dxk[0, 0, :]; dxk = dxk[:, 1, :]
pk = dpk[0, 0, :]; dpk = dpk[:, 1, :]
foo = lambda t: np.linalg.det(jshoot(tc[k], xk, pk, t, dxk, dpk))
sol = nle.solve(foo, tc[k], options=nleopt); tc[k] = sol.x
ax21.plot(tc[k], 0, 'r*')
ax22.plot(tc[k], 0, 'r*')
print('tc =', tc)
Calls |f(x)| |x|
1 7.452820308014326e+02 5.219371301468111e+02
2 7.499244354398687e+01 5.220844406941543e+02
3 5.854295671065765e+00 5.220709730339374e+02
4 3.568146879603087e-02 5.220719482577457e+02
5 1.742250554656049e-05 5.220719542381074e+02
6 1.868184367148713e-10 5.220719542351887e+02
Results of the nle solver method:
xsol = 522.0719542351887
f(xsol) = -1.868184367148713e-10
nfev = 6
njev = 1
status = 1
success = True
Successfully completed: relative error between two consecutive iterates is at most TolX.
Calls |f(x)| |x|
1 5.839098509598629e+03 6.836880670878310e+02
2 7.635989933122853e+02 6.837171213473797e+02
3 1.202551425483022e+02 6.837214925040061e+02
4 3.149560057372592e+00 6.837223095695031e+02
5 1.348484495495010e-02 6.837223315445174e+02
6 1.606592157326722e-06 6.837223316390080e+02
Results of the nle solver method:
xsol = 683.722331639008
f(xsol) = 1.606592157326722e-06
nfev = 6
njev = 1
status = 1
success = True
Successfully completed: relative error between two consecutive iterates is at most TolX.
tc = [522.07195424 683.72233164]